Solar energy

Author

Affiliation

Beniamino Sartini

University of Bologna

Published

May 1, 2024

Modified

June 30, 2024

The sun is the main source of renewable energy available on the earth. We define as solar energy every form of energy directly derived from the sun, i.e. the photovoltaic one. However, the other renewable sources are also indirectly connected with the sun. Hydraulic energy, for example, is linked to the water cycle in nature through evaporation and the subsequent precipitation, which is caused by solar heating. Similarly, the winds, from which wind energy derives, are generated by changes in atmospheric pressure linked to solar heating. Finally, also the chemical potential energy of biomass is a form of solar energy storage captured by plants through photosynthesis. From this point of view, it is legitimate to say that fossil fuels also owe their existence to the sun, as they were formed over the geological eras by the anaerobic transformation of organic substances Comini and Savino (2013). It is therefore clear how all the forms of energy that we have at out disposal are directly or indirectly connected to the presence and energy of the sun. This chapter is devoted to understanding the nature of the energy irradiated by the sun and its movements. The first section is devoted to the solar geometry, in which we will go through the definitions of the angles that characterize the position of the sun with respect to a point on the earth and the direction and angle of incidence of a solar solar ray. In the second section we will examine the characteristics of the extraterrestrial radiation: it is important in understanding and using solar radiation data, since it should represent an upper limit of the amount of solar radiation available on the earth. More details on these arguments can be found in Duffie and Beckman (2013).

1 The Sun

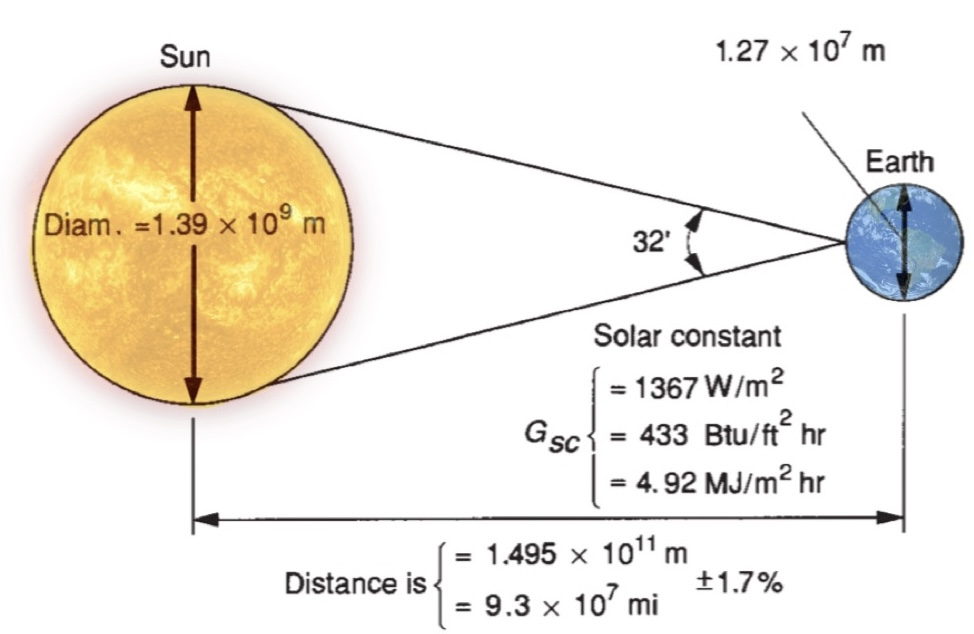
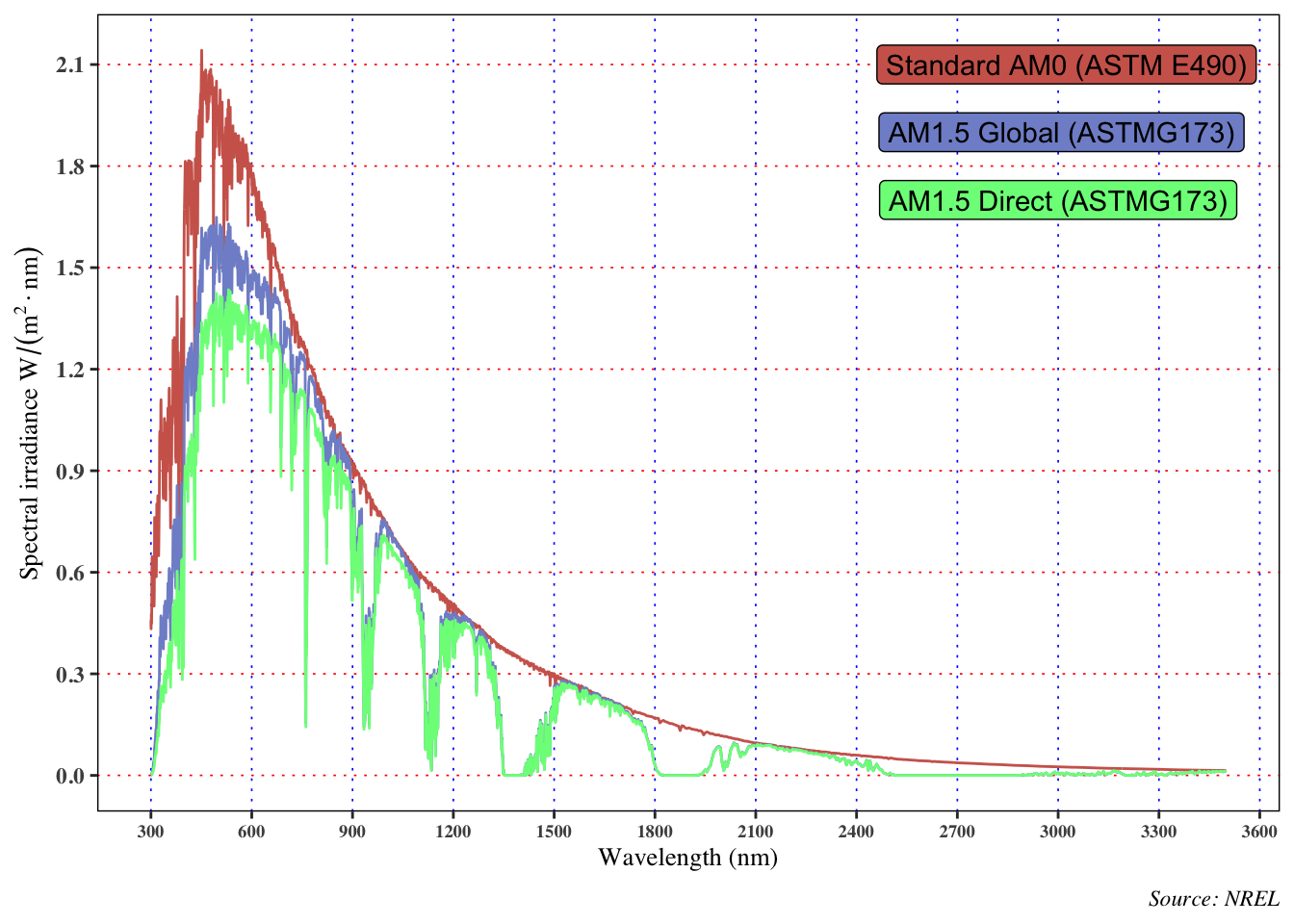
The sun is a sphere with a diameter of 1.39 x $10^{9}$ m and on average its distance from the earth is $1.5 \times 10^{11} m$. Seen from the earth it rotates on its axis every 4 weeks, however the rotation is not perceived equal from all the point of the earth but is about 27 days at the equator and 30 days on polar regions. The sun has an effective black body temperature¹ of 5777 K, but on its interior the temperature is much higher, then the energy produced inside is transferred to the surface and irradiated into space till the earth.

However, in reality the sun does not work, as a black body radiator at a fixed temperature, rather the emitted solar radiation is the composite result of the several layers that emit and absorb radiation of various wavelengths. The final extraterrestrial solar radiation and its spectral distribution have now been measured by various methods in several experiments. The solar spectrum changes throughout the day and with location. Standard reference spectra are defined to allow the performance comparison of photovoltaic devices from different manufacturers and research laboratories. We can distinguish between three main standards:

AM0: is the standard spectrum for space applications, it has an integrated power of $1366.1 \; W/m^2$.
AM1.5 Global spectrum: is designed for flat plate modules and has an integrated power of $1000 \; W/m^2$.
AM1.5 Direct spectrum: is defined for solar concentrator work, it includes the direct beam from the sun plus the circumsolar component in a disk 2.5 degrees around the sun. The direct plus circumsolar spectrum has an integrated power density of $900 \; W/m^2$.

The data for these three spectra are available for the download at the website². Assuming that the sun behaves as a blackbody, we could compare these spectra with the theoretical distribution obtained by the Planck’s law. Denoting as $T = 5777 \; K$ the temperature of the sun, with $R_s = 6.955 \cdot 10^{8} \; m$ its radius and with $R_{se} = 1.495 \cdot 10^{11} \; m$ the mean distance sun-earth, then the Planck’s Law allow to write the emittivity power of the sun as:

\[ E_{\lambda} = \left( \frac{R_{s}}{R_{se}} \right)^{2} \frac{C_1}{\lambda^{5} [\exp(C_{2}/\lambda T)-1]} \tag{1}\]

In Equation 1, $\lambda$ is the wavelength expressed in micrometers, $C_1$ and $C_2$ are two constants given by

\[ C_1 = 3.742 \cdot 10^8 \; (W \mu^4)/m^2 \quad\quad C_2 = 1.439 \cdot 10^4 \; \mu K \]

As consequence $E_{\lambda}$ will be measured in $[W/(m^2 \mu m)]$. In Figure Figure 2, the four spectra are compared: it is interesting to note the differences among the red and the blue and green curves, that represent the real and the adjusted spectral distribution of the sun, respectively. The air mass zero (AM0), or extraterrestrial spectrum used to generate the terrestrial reference spectra was developed by Gueymard Gueymard (2004) and is a synthesis of several air mass zero data sets. The comparison between AM0 and AM1 highlights by difference the effects of atmospheric attenuation on the direct component arriving on a surface orthogonal to the sun’s rays. These effects are particularly accentuated in the absorption bands of ozone (300-400 $nm$), water vapor (800-2000 $nm$) and carbon dioxide (above 2500 $nm$).

Figure 2: Spectal distribution of the sun under different standards (Source: NREL).

1.1 Radiation Definitions

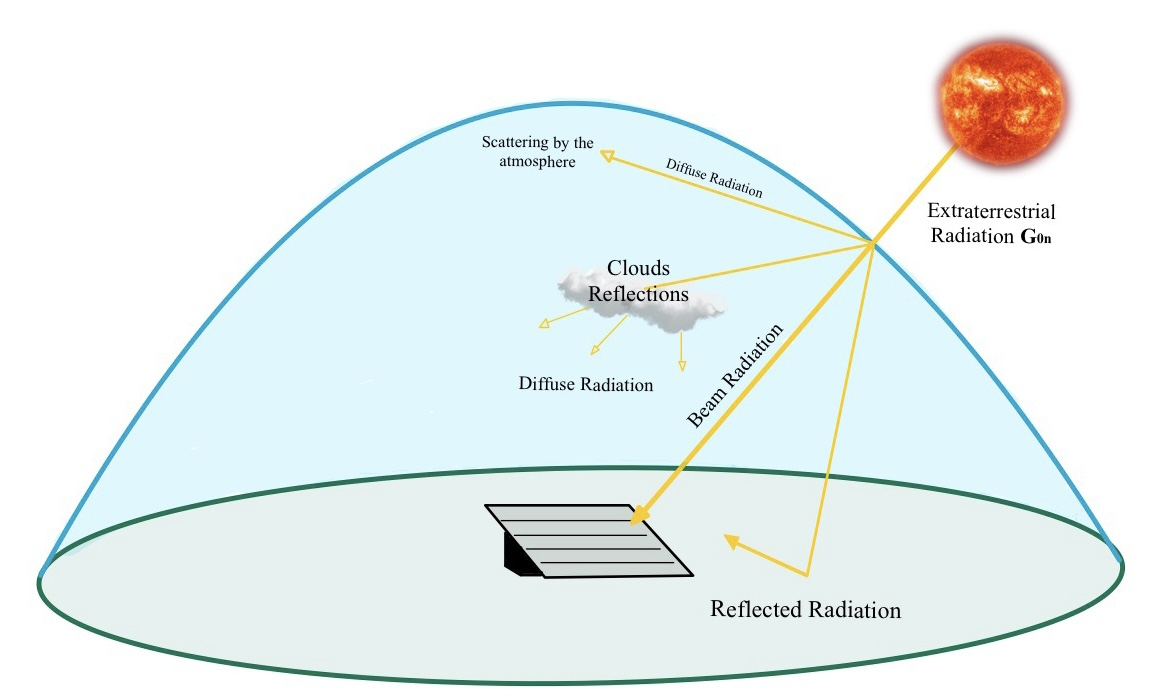
As the solar radiation goes through the atmosphere it suffers different processes of absorption, dispersion or scattering that result in lower levels of solar radiation being received at the Earth’s surface. These are due to the atmosphere components, such as ozone or $CO_2$, and solid and liquid particles in suspension like aerosols or water vapor. However, the main source of attenuation is the cloud cover. In general, the processes of absorption and attenuation differently affect the wavelengths of solar radiation, therefore the spectral distribution of the solar radiation at ground level differs from the extraterrestrial one. We can distinguish between four quantities that in general are used to express solar radiation:

Extraterrestrial Radiation: it represents the amount of radiation that could be theoretically available if there were no atmosphere. Even if it could present some randomness, in general it is considered deterministic. Once the radiation enters in the atmosphere we could make two further distinctions in beam and diffuse radiation. The notations involved are several: $G_{sc}$ is the solar constant, $G_n$ is the value of $G_{sc}$ corrected for the $n$-th day of the year. Moreover, $G_{0,n}$ represents $G_{n}$ computed for an horizontal surface. Finally $H_{0,n}$ is the daily integral of $G_{0,n}$. More details will be given below.
Beam Radiation: it represents the solar radiation received from the sun without being scattered by the atmosphere. Consequently to its definition, the Beam radiation is maximum when the sky is clear³. In theory the Beam radiation can be zero: even if this could seem strange, in days when the sky is fully covered by clouds, the instruments devoted to the measurement⁴ could register a value of zero. It is also denoted as Direct Normal Irradiance or DNI.
Diffuse Radiation: it represents the solar radiation received from the sun after its direction has been changed and scattered by the atmosphere. In application, usually, this measure is considered with respect to an horizontal surface (Diffuse Horizontal Irradiance or DHI).
Global Solar Radiation: it represents the sum of the beam and the diffuse radiations. A common practice is to consider it with respect to an horizontal surface (Global Horizontal Irradiance or GHI).

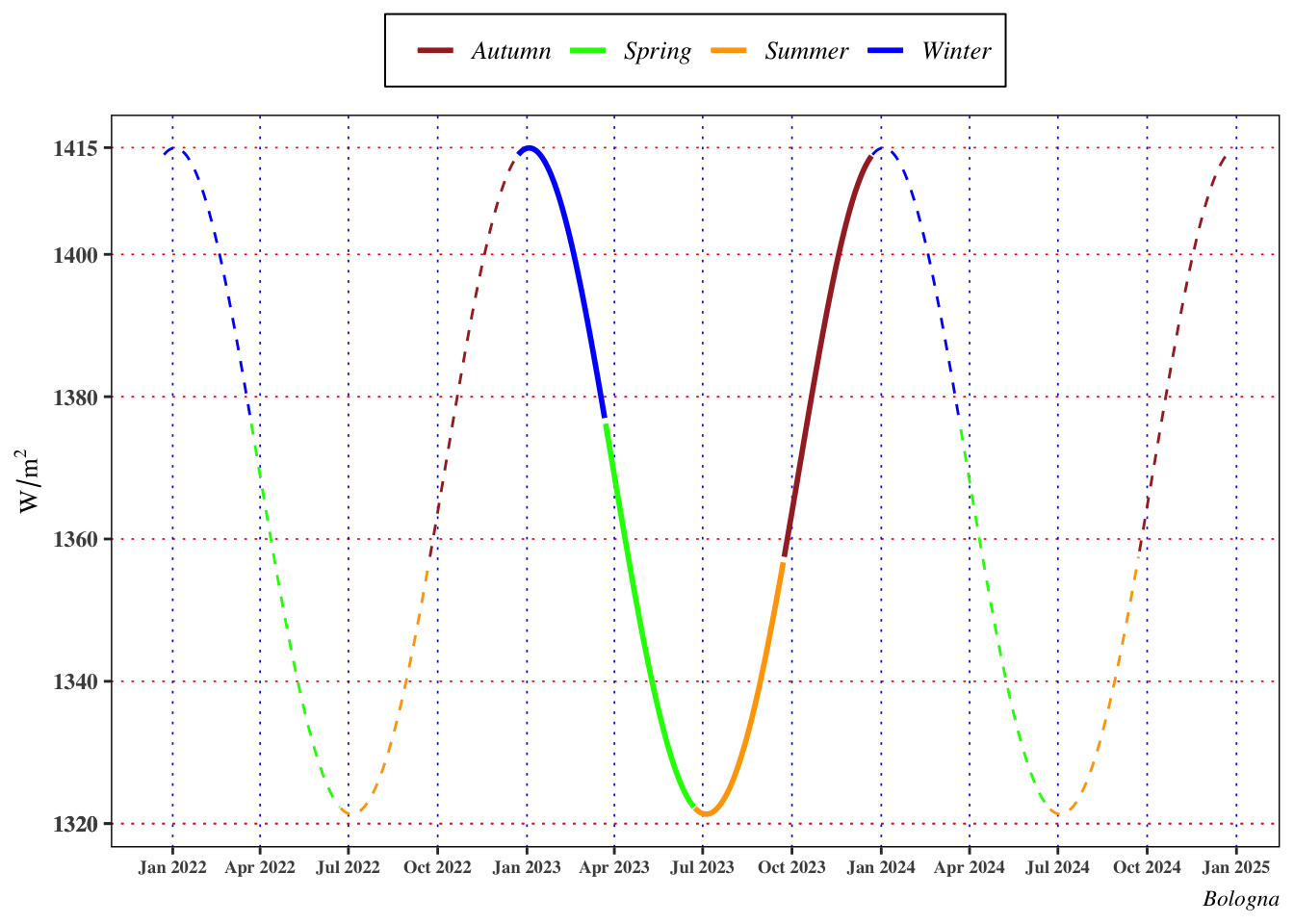
Figure 3: Different definitions types of solar irradiance.

1.2 Solar constant

The solar constant $G_{sc}$ is defined as the energy received from the sun per unit of time (typically a second), on a unit area of surface perpendicular to the direction of propagation of the radiation at the mean earth-sun distance outside the atmosphere. The most recent estimate from the Wold Radiation Center in Switzerland gives a mean value of $\bar{G}_{sc} = 1367 \, W/m^2$. In order to prove this result, we have to consider the total energy irradiated from the sun per $m^2$, given by: $q_0 = 6.316 \cdot 10^7 W/m^2$. Denoting as $R_s$ the radius of the sun and with $R_{se}$ the mean earth-sun distance, the following simple equation with $G_{sc}$ unknown can be solved:

\[ q_{0} 4 \pi R_{s}^2 = G_{sc} 4 \pi R_{se}^2 \; \Longrightarrow \; G_{sc} = q_{0} \frac{R_{s}^2}{R_{se}^2} = 1367 \, W/m^2 \tag{2}\]

However, $G_{sc}$ is actually not constant. For practical purposes it is possible to neglect the variations due to sunspots⁵ and to assume that the flow of energy radiated by the sun, $q_0$, is constant. Consequently, $G_{sc}$ will change according to the variation of the earth-sun distance. Counter intuitively the solar constant assumes its maximum value (around 1420 $W/m^2$) during the lasts days of December and its minimum (around 1320 $W/m^2$) during the summer in the late June. Spencer (1971), as cited by Iqbal Iqbal (1983), recovered an accurate equation ($\pm 0.01 \%$) that can be used for the correction of $G_{sc}$ during the year. Denoting as $G_{n}$ the extraterrestrial radiation incident on the plane normal to the radiation on the $n$-th day of the year:

\[ G_{n} = G_{sc} ({\small{1.000110}} + {\small{0.034221}} \cos B + {\small{0.001280}} \sin B + {\small{0.000719}} \cos 2B + {\small{0.00077}} \sin 2B) \text{,} \tag{3}\] where $B$ represents an angle in radiant i.e. \[ B = (n-1) \frac{2 \pi}{365} \text{.} \tag{4}\]

Figure 4: Solar constant computed in Bologna.

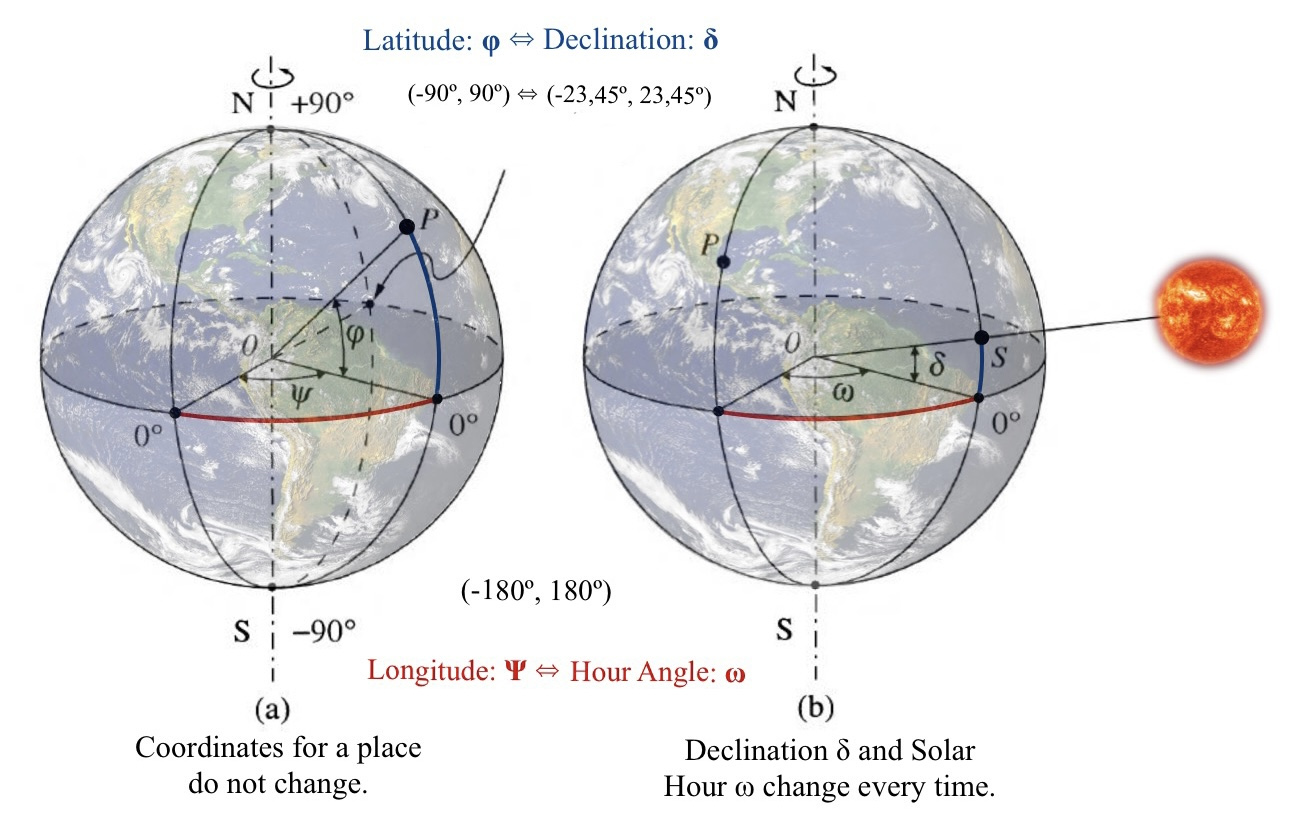
1.3 Equatorial coordinates

The position of the sun with respect to a point $P$ on the Earth’s surface can be identified through an equatorial coordinate system. Each point on the Earth’s surface is identified by a pair of angular coordinates:

Figure 5: Relations between declination and latitude, and hour angle and longitude.

Latitude $\phi$: it is the angular location at the north or at the south of the equator, where by convention it is considered a positive sign in the north with respect to the equator and negative in the south. It varies in the range [-90°,90°]. In practice, it is the angular distance of $P$ from the equator measured along the meridian passing through $P$.
Longitude $\psi$: it is the angular distance, measured along the equator, of the meridian passing through $P$ from the meridian of Greenwich $G$. It is considered positive at the East of $G$ and negative at West. It varies in the range [-180°,180°]. Based on the definitions, latitude and longitude are equatorial coordinates: they refer exclusively to the equatorial plane and to the Earth’s axis⁶. By tracing the junction between the center of the Earth and that of the sun, it is possible to find the point of intersection S. In this context we define:
Solar Declination $\delta$: homologous of latitude, it is the angular position of the sun when it is situated on the local meridian with respect to the plane of the equator. As the latitude is positive in the North and negative in the South. It varies in the range [-23.50°,23.50°]. By definition it is equal for every point on the Earth.
Hour Angle $\omega$: homologous of longitude, it is the angular displacement of the sun at the East or West of the local meridian due to the rotation of the Earth on its axis. It is negative in the morning, zero at the solar noon and positive in the afternoon. It is defined as the difference between the longitude of $P$ (fixed) and the longitude of $S$ (variable). It is equal for all the points on the same meridian.

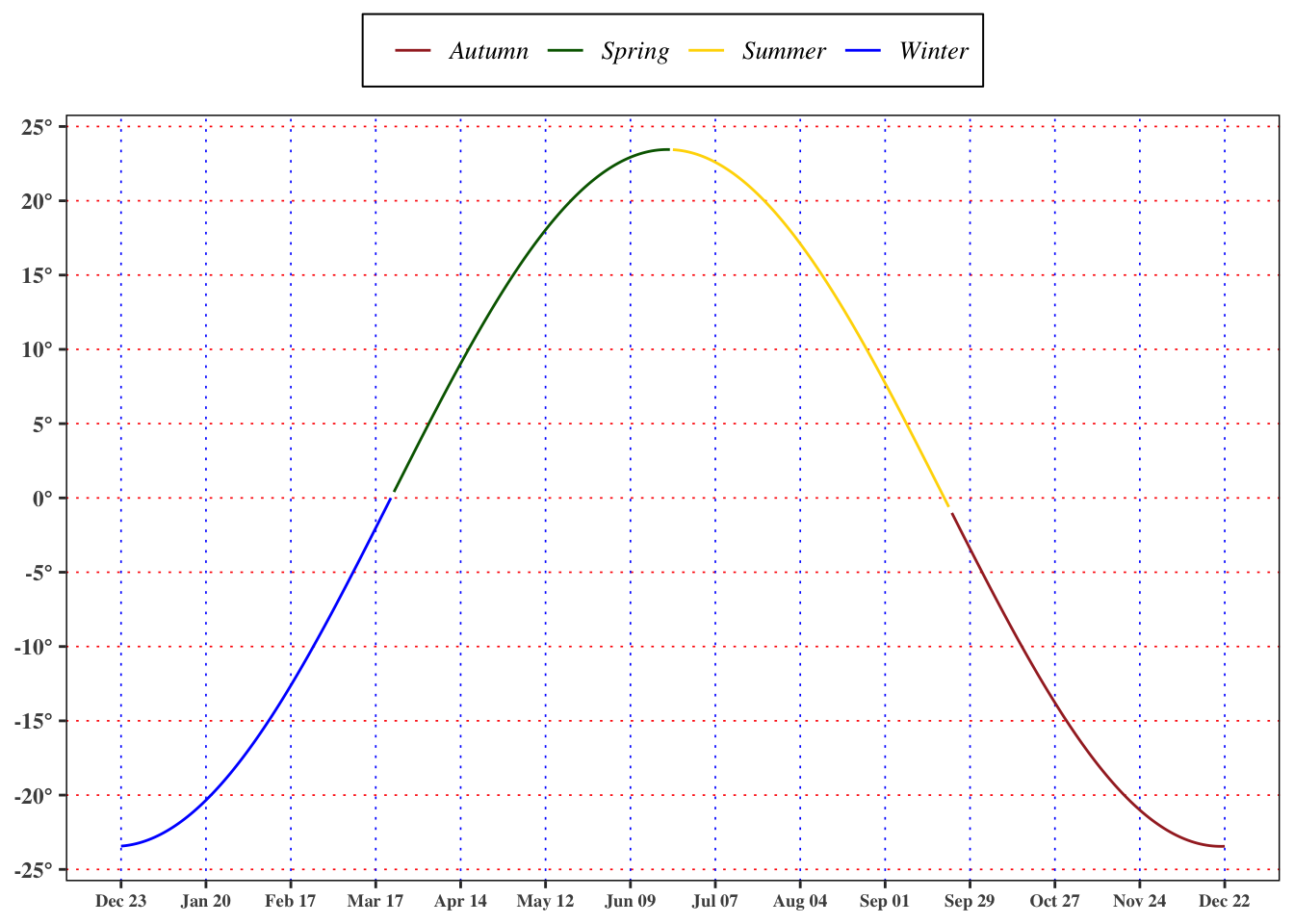
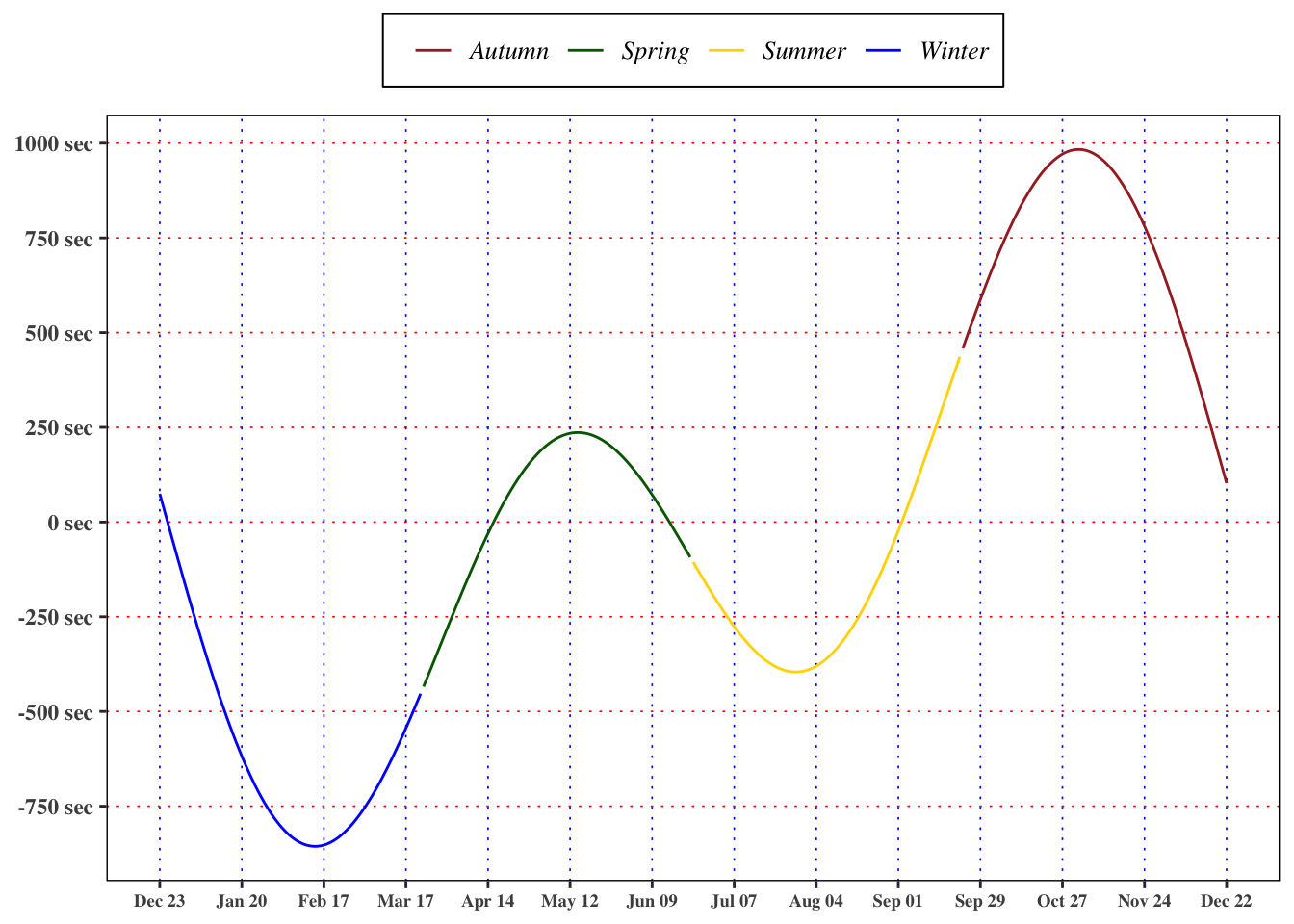
1.3.1 Solar declination $\delta$

Given $n$, that represents the number of the day during the year, the solar declination can be approximated with a well known equation proposed by Cooper Cooper (1969):

\[ \delta = 23.45 \sin \left( 360 \frac{284+n}{365} \right) \tag{5}\]

In 1971, Spencer, as cited by Iqbal (1983), found a more accurate specification, that gives an error less $\underline{+} 0.035°\%$.

\[ \begin{split} \delta = \frac{180}{\pi} \bigl( & {\small{0.006918}} - {\small{0.399912}} \cos B + {\small{0.070257}} \sin B - {\small{0.006758}} \cos 2B + \\ & + {\small{0.000907}} \sin2B - {\small{0.002697}} \cos3B + {\small{0.00148}} \sin3B \bigl) \end{split} \tag{6}\]

where $B$ is the same as Equation 4. During the revolving movement, the Earth’s axis is constantly inclined towards the North Star at an angle of 23.45 with respect to the plane of the orbit. This inclination is responsible for the variation of the solar constant during the year and consequently for the number of hours per day and the changing of the seasons.

1.3.2 Hour angle $\omega$

During a movement of rotation of the Earth around its axes the hour angle $\omega$ changes of 360°. Therefore in a day of around 24 hours the hour angle vary of 15° degrees each hour. However the duration of the mean day is not exactly 24 hours, given the clock hour $\overline{\theta}$ (eventually without the Legal Hour) the relation with the solar hour $\theta_s$ is the following: \[ \theta_s - \overline{\theta} = 4 \, \bigl( \, \psi_P - \psi_{ref} \, \bigl) + E_{n} \] The difference between the two hours is expressed in minutes. $\psi_P$ represents the longitudes of the meridian of the observer, while $\psi_{ref}$ denote the longitude of the meridian where the local time zone is settled (for Italy and Central EU the reference is $\psi_{ref} = 15$°). The last element $E(n)$ represents a time correction in minutes:

\[ E_{n} = {\small{229.2}} ({\small{0.00075}} + {\small{0.001868}} \cos B - {\small{0.032077}} \sin B - {\small{0.014615}} \cos 2B - {\small{0.04089}} \sin 2B) \tag{7}\]

where $n$ is the number of the day and $B$ is the same as Equation 4. Once that the solar hour is known, it is possible to recover the hour angle $\omega$ as:

\[ \omega = \psi_{ref} \, (\theta_s - 12) \] where $\theta_s$ is expressed in hours.

Figure 7: Seasonal time adjustment in Bologna.

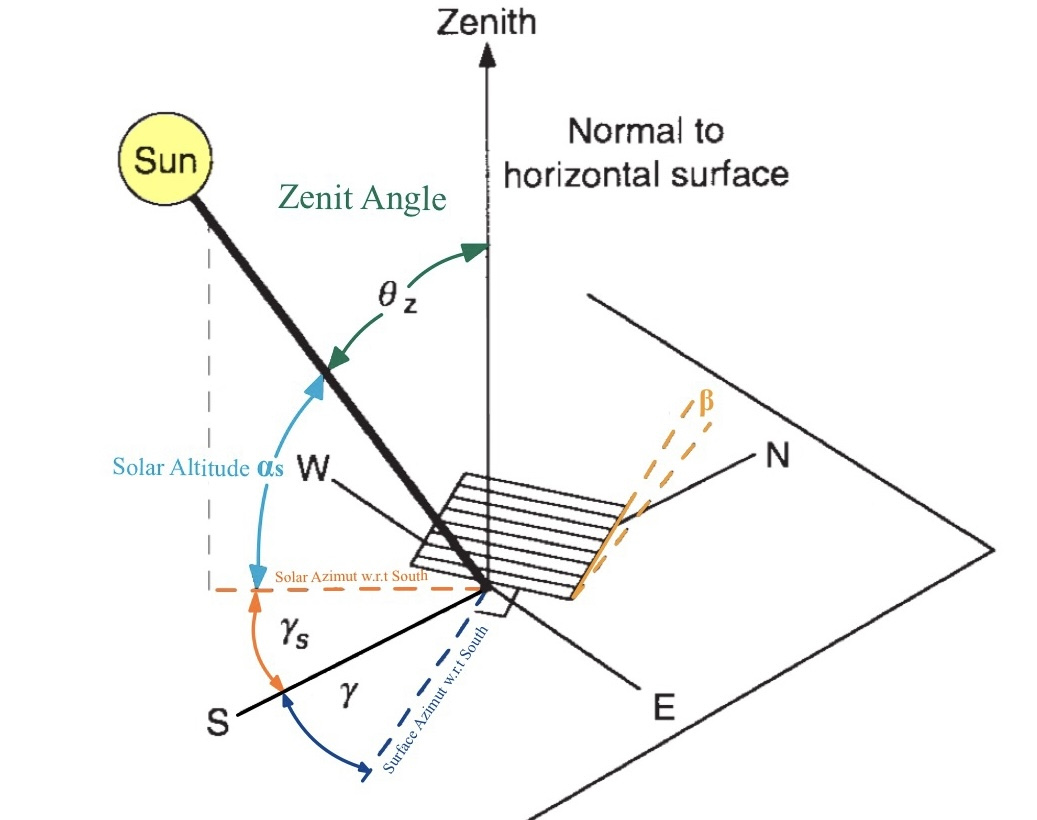
1.4 Terrestial Coordinates

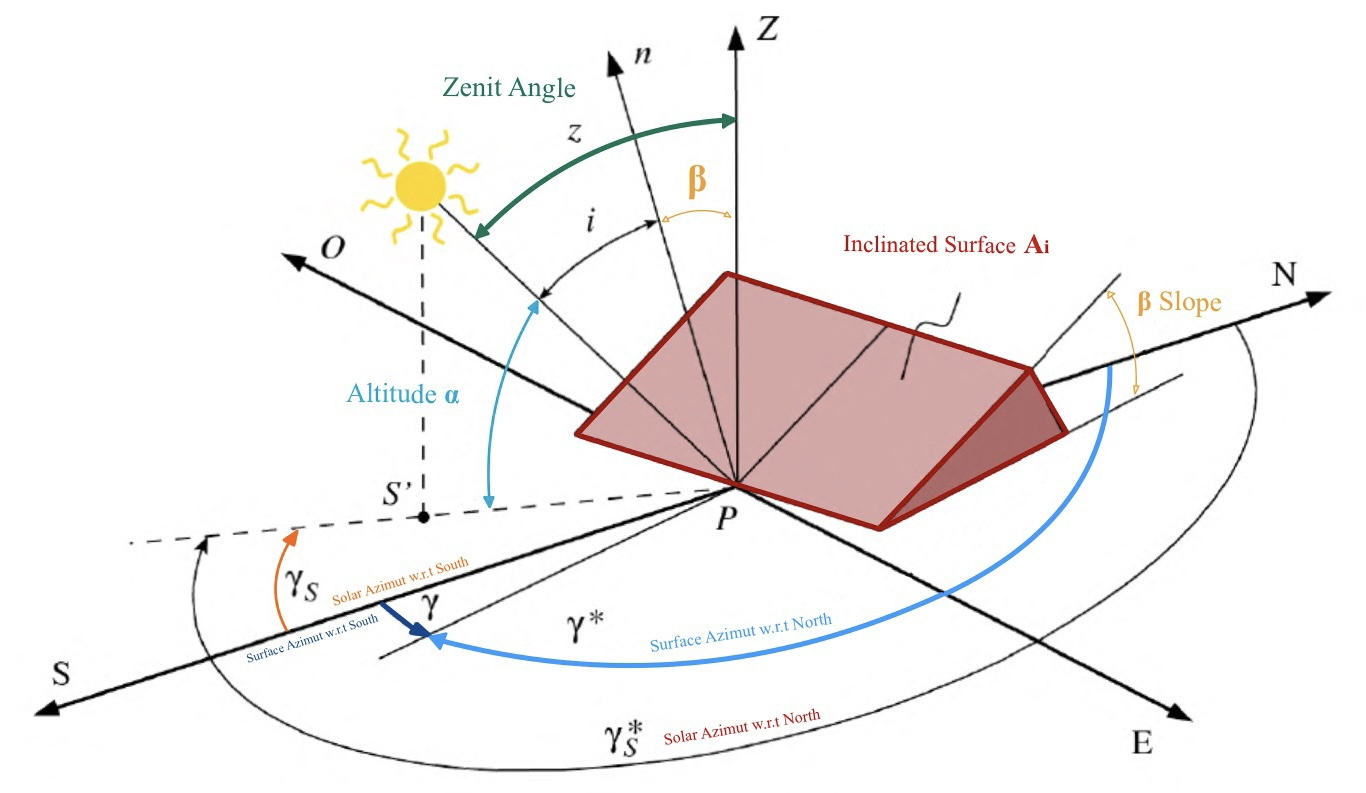
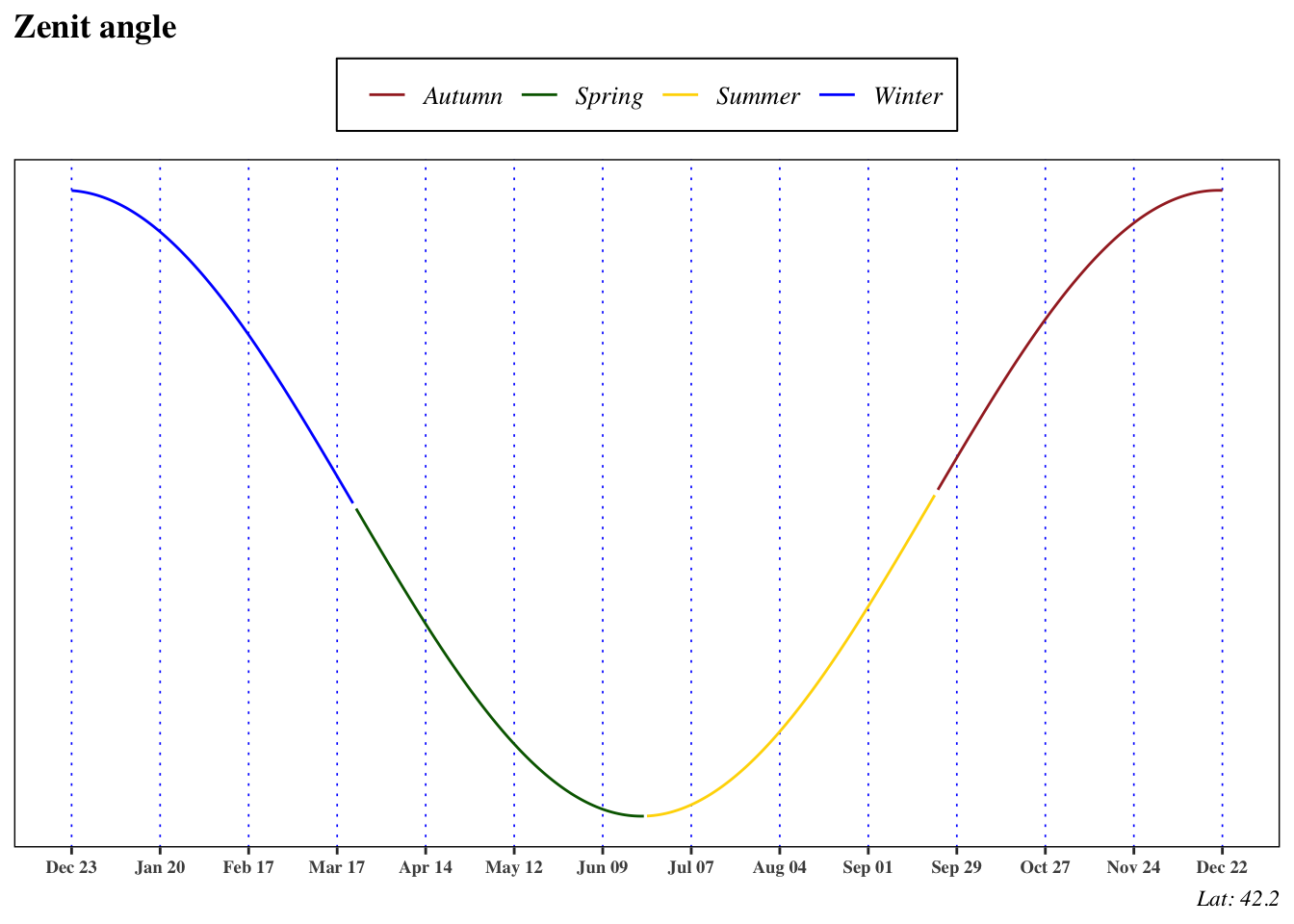
The irradiance of a receiving surface depends on the cosine of the angle of incidence, i.e. the angle that the direction of the sunrays forms with the normal $n$ at the surface itself. The variations of angle of incidence over time are the result of the rotational and revolutionary movements of the earth with respect to the sun. An alternative reppresentation of the equatorial coordinates is given by the terrestrial coordinates⁷. In solar engineering often this different system is used because it is more intuitive and easier to visualize. The geometric relationships between a plane of any particular orientation relative to the earth at any time and the incoming beam solar radiation ⁸, can be described in terms of several angles Benford and Bock (1939).

Figure 8: Position of the sun in terrestrial coordinates.

Zenith angle $\theta_z$: it is the angle between the vertical line and the line to the sun, it represents the angle of incidence of the radiation on an horizontal surface.
Solar altitude angle $\alpha_s$: it is the angle between the horizontal line and the line of the sun and can be defined as the complement of the zenit angle.
Solar azimut angle $\gamma_s$: it is the angular displacement from the south of the projection of the radiation on the horizontal plane.
Angle of incidence $\theta$: it is the angle formed by the Beam radiation on a surface and the normal to that surface. Considering an horizontal receiving surface the angle of incidence is actually equal to the zenith angle. If we consider an horizontal surface, the previous angles are the only ones needed for the computation of the incidence angle of the beam radiation. However it is possible to consider a more general relation that takes into account also the orientation and inclination of a surface three more angles can be defined:
Slope $\beta$: it is the angle between the plane of the surface and the horizontal. It varies in the range [0°, 180°].
Angle of incidence $\theta$: it is the angle formed by the beam radiation on a surface and the normal to that surface.
Surface azimut angle $\gamma$: it represents the deviation of the projection on an horizontal plane of the normal to the surface from the local meridian. It is considered zero at the South, negative in the East and positive in the West.

Figure 9: Angular Relationships for an inclinated surface.

There is a set of relations that connect the angle of incidence to the others that are time varying: \[ \cos \theta = T + U \cos \omega + V \sin \omega \tag{8}\] \[ \cos \theta = \cos\theta_z \cos\beta + \sin\theta_z \sin\beta \cos(\gamma_s - \gamma) \tag{9}\] where $T$, $U$, $V$, are three coefficients that can be computed as: \[ \begin{cases} T = \sin\delta (\sin\phi \cos\beta - \cos\phi \sin\beta \cos\gamma) \\ U = \cos\delta (\cos\phi \cos\beta - \sin\phi \sin\beta \cos\gamma ) \\ V = \cos\delta \sin \beta \sin \gamma \end{cases} \] The relations Equation 8 and Equation 9 are equivalent and each one can be used to determine the $cos \theta$, the only difference is in the required inputs. They can be applied for every oriented and inclined surface, however there are two special cases that are convenient to analyze. The first one is when we consider a surface oriented at south, in this case $\gamma = 0$ and we are able to simplify the relation Equation 8 as: \[ \cos \theta = \sin\delta \sin(\phi - \beta) + \cos\omega \cos\delta \cos(\phi - \beta) \tag{10}\] The second special case is for an horizontal surface, in this case there is no inclination and the orientations are unconcerned, therefore $\beta = 0$ implies that $\gamma$ will no more enter in the formula: \[ \cos\theta = \cos\theta_z = \sin\delta \sin\phi + \cos\delta \cos\phi \cos\omega \tag{11}\] Moreover in the case Equation 11, the zenit angle $\theta_z$ is equal to the incidence angle $\theta$. In general this is not true, and $\theta$ can be recovered given $\theta_z$ and the other solar angles and applying the Equation 8 or Equation 9. As consequence of the relation Equation 11 there is the possibility to recover the hour angle at the sunrise and at the sunset, $\omega_s$. In these two particular moments of the day we have that $\theta_z = 0$, and applying the formulas of $\omega_s$ for that day is given by: \[ |\omega_s| = \pm \cos^{-1} (-\tan \delta \tan\phi) \tag{12}\] Considering an horizontal surface the sunset and sunrise hour angles are equal and opposite, in general this is not true for an oriented and inclined surface. Knowing the value of $\omega_s$ allow to know if in a certain time of the day the sun will be visible or not in the sky, therefore we can establish also the maximum amount of sun hours for an horizontal surface which is obtained dividing the degree routes of the sun by the time spent (in minutes) to move one degree⁹. \[ \text{Hsun} = \frac{2 |\omega_s|}{15} \tag{13}\]

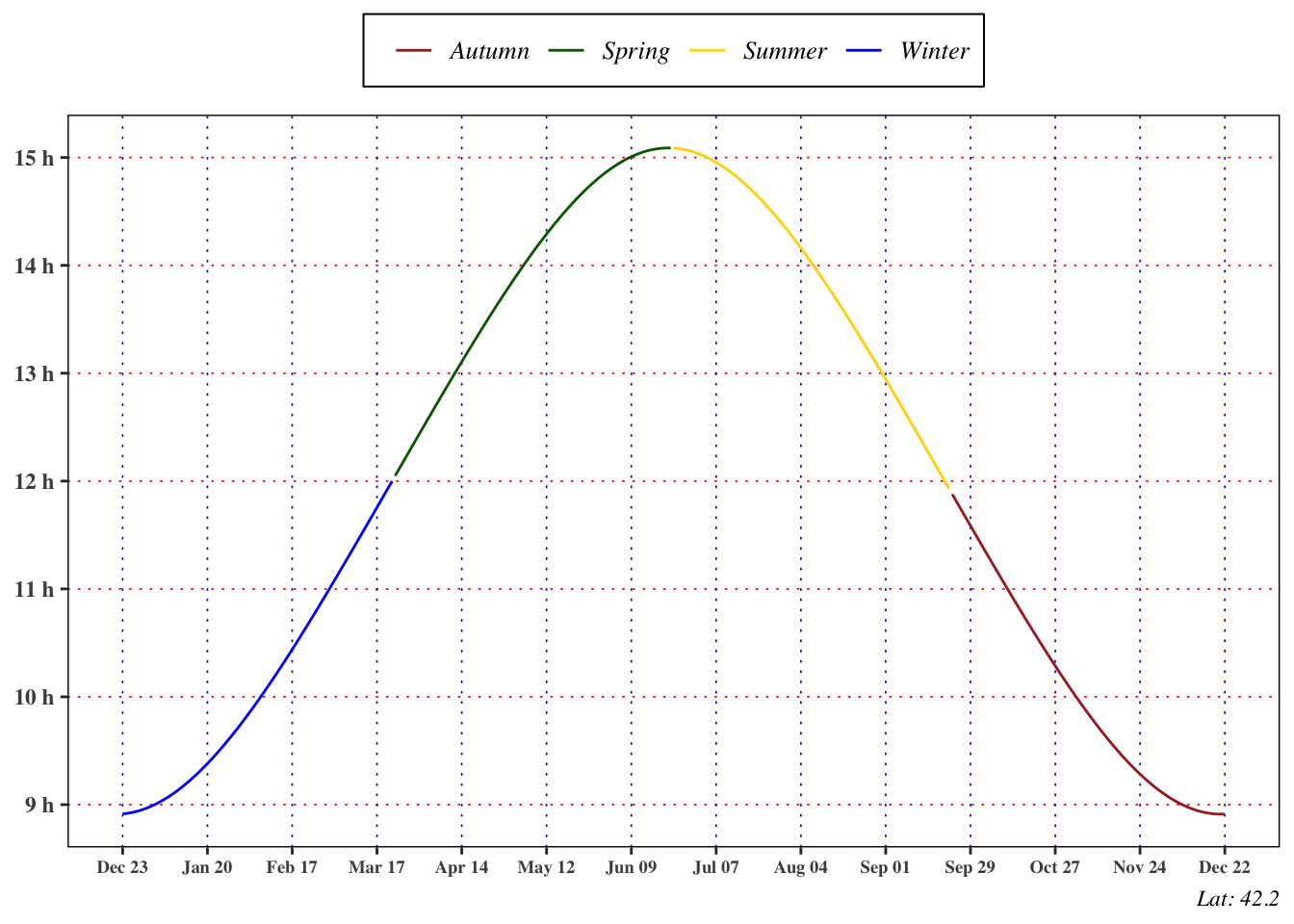
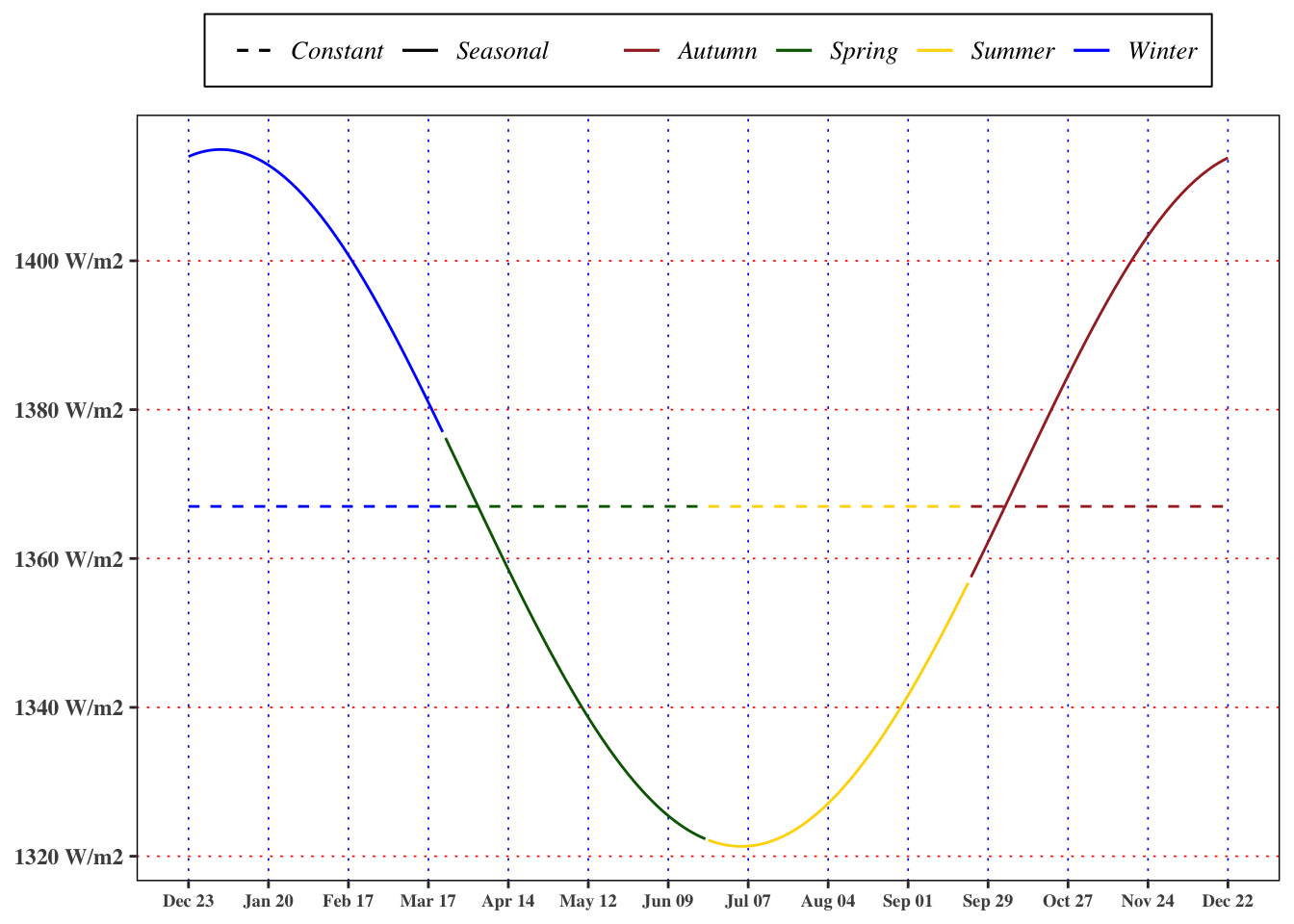
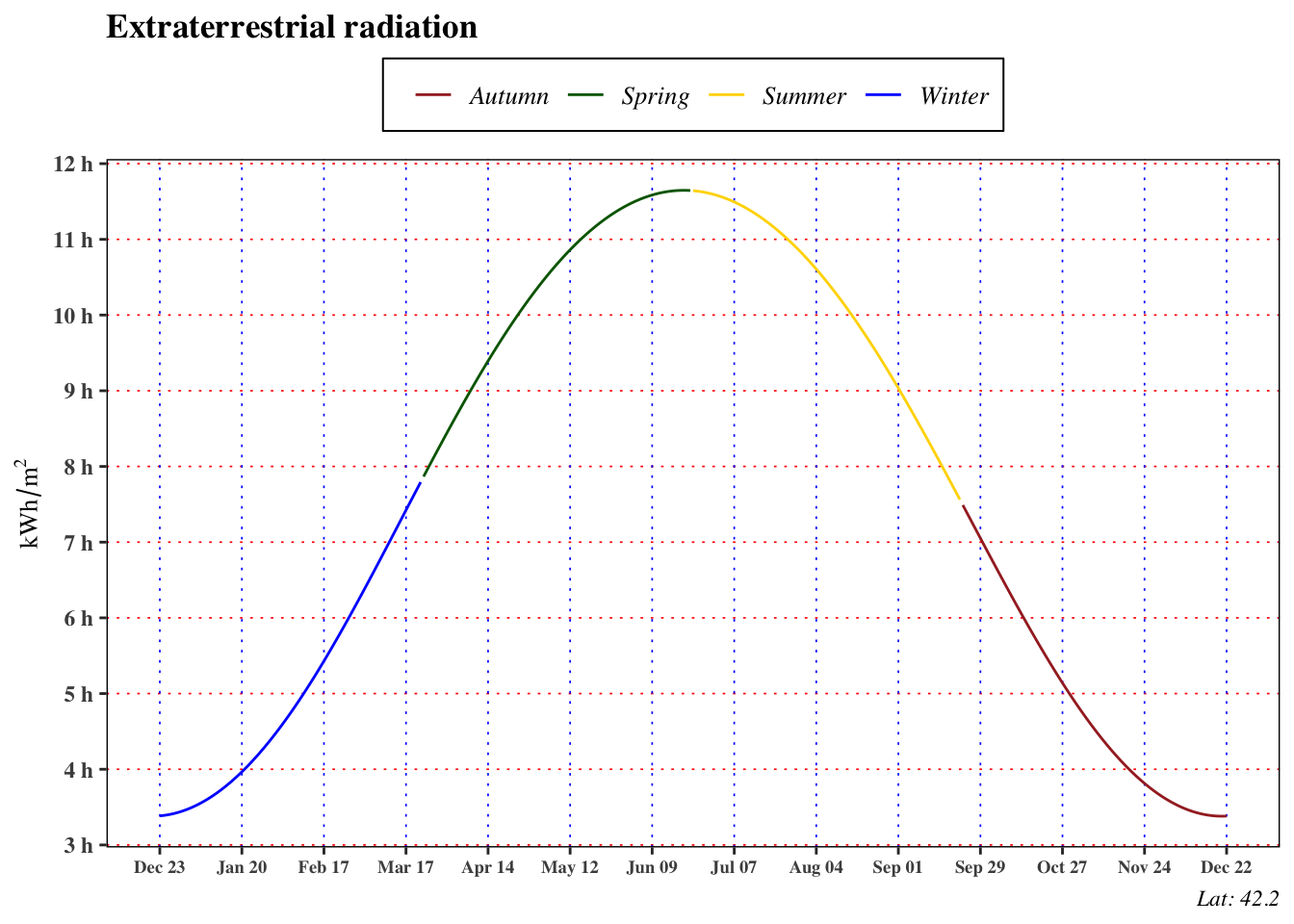
1.5 Extraterrestrial Radiation

Considering the variation of the extraterrestrial radiation during the year, there are two sources of stochasticity. The first is given by a variation of the radiation emitted by the sun, in literature there are conflicting reports on periodic variations of intrinsic solar radiation. For engineering and modelling purposes this source of stochasticity is neglected. However the second source of variability is given by the different earth-sun distance during the year that leads to difference of $\pm 3.3 \%$. At any point in time, the solar radiation incident on a horizontal plane outside the atmosphere can be computed multiplying the cosine of the angle of incidence $\theta_z$ by the solar constant as computed in Equation 2. \[ G_{0,n} = G_{n} \cos \theta_z = G_{n} (\cos\delta \cos\phi \cos\omega + \sin\delta \sin\phi) \tag{14}\]

Figure 12: Seasonal solar constant $G_{0,n}$ and $G_0$ in Bologna.

This expression represents the extraterrestrial energy that arrives on an horizontal surface in any time between the sunrise and the sunset. A more useful expression in the application is the daily extraterrestrial horizontal irradiance, denoted as $ H_{n}^{extra}$, that can be computed integrating the formula Equation 14 with respect to $\omega$, i.e. \[ H_{n}^{extra} = G_{n} \frac{24 \times 3600}{\pi} \left(\cos\delta \cos\phi \sin\omega_s + \frac{\pi \omega_s}{180} \sin\delta \sin\phi\right) \tag{15}\] If $G_{sc}$ is expressed as $W/m^2$, then $H_{n}^{extra}$ as computed in Equation 15 will be expressed in $J/m^2/day$. Another very useful relation is given by the hourly extraterrestrial daily irradiance that can be computed as for a period between two hour angles, with $\omega_2 > \omega_1$:
\[ H_{n}^{\omega_1,\omega_2} = G_{n} \frac{12 \times 3600}{\pi} \left(\cos\delta \cos\phi \left(\sin\omega_2 - \sin\omega_1\right) + \frac{\pi (\omega_2 -\omega_1)} {180} \sin\delta \sin\phi\right) \tag{16}\]

Figure 13: Solar extraterrestrial radiation in Bologna.

References

Benford, F., and J. E. Bock. 1939. “A Time Analysis of Sunshine.” Trans. Am. Illumin. Eng. Soc. 34: 200.

Comini, G., and S. Savino. 2013. La Captazione Dell’energia Solare. CISM.

Cooper, P. I. 1969. “The Absorption of Radiation in Solar Stills.” Solar Energy 12 (3): 333–46. https://doi.org/10.1016/0038-092X(69)90047-4.

Duffie, John A., and William A. Beckman. 2013. Solar Engineering of Thermal Processes. New York: John Wiley; Sons.

Gueymard, C. 2004. “The Sun’s Total and Spectral Irradiance for Solar Energy Applications and Solar Radiation Models.” Solar Energy 76 (4): 423–53.

Iqbal, M. 1983. An Introduction to Solar Radiation. Elsevier.

Footnotes

The effective black body temperature of 5777 K is the temperature of a black body radiating the same amount of energy as does the sun.↩︎
https://www.nrel.gov/grid/solar-resource/spectra-am1.5.html ↩︎
Clear sky is the condition where the visibility is greater or equal to 23km.↩︎
Pyrheliometers are used for the measure of Beam normal radiation, while pyrometers are used for the measure of the global radiation on horizontal surface↩︎
Areas of the sun where the temperature is about 5000 K.↩︎
As is known, the Earth’s axis intersects orthogonally the equatorial plane at the point O, center of the Earth.↩︎
Terrestrial coordinates are centered on a ground surface containing the location P of the observer.↩︎
i.e., the position of the sun relative to that plane↩︎
For Europe the time occurred to move one degree is around 15 minutes.↩︎

Citation

BibTeX citation:

@online{sartini2024,
  author = {Sartini, Beniamino},
  title = {Solar Energy},
  date = {2024-05-01},
  url = {https://greenfin.it/solar/solar-energy.html},
  langid = {en}
}

For attribution, please cite this work as:

Sartini, Beniamino. 2024. “Solar Energy.” May 1, 2024. https://greenfin.it/solar/solar-energy.html.